
Rod Lakes, University of Wisconsin, Engineering Mechanics and Rheology Research Center |

Rod Lakes, University of Wisconsin, Engineering Mechanics and Rheology Research Center |
Viscoelasticity: creep
This animation shows creep of a rod (60 cm long, 3.26 mm diameter) of polymethyl methacrylate (PMMA) in cantilever bending at room temperature, ~20 deg. C. The initial droop of the rod under its own weight was 84 mm. Larger image.
Images of the free rod end were captured after ~1, 10, 100, 1,000, 104, 105 seconds, 106 seconds (about 11 days), 107 seconds (almost four months). The images were captured at equal intervals on a log time scale; they are presented at equal intervals in a linear time scale. In viscoelasticity, think logarithmically! If this creep were viewed in linear time, you might think it had reached an asymptote after a few minutes. Actually, based on time-temperature superposition, one expects the creep to continue for millions of years. Compare with a single exponential relaxation which covers only about one decade (a factor of ten).
Viscoelasticity: damping

To illustrate effects of material damping (a manifestation of behavior variously called viscoelasticity, anelastic behavior, internal friction, hysteresis), listen to the sound of tuning forks with different damping. Each fork is identical in size and shape, but the material stiffness and density are different, giving rise to differences in pitch. The number n of cycles for the vibration to decay by a factor e = 2.718.. is related to damping tan delta by tan delta = 1/np.
The delta in tan delta is the phase angle between stress and strain.
This phase is usually represented as a lower case Greek delta. If your web browser does not interpret Symbol font properly, d may instead look like a bold face d. Similarly Greek pi may instead look like a bold face p.
Aluminum alloy, tan delta less than 0.0002, inferred from the decay of vibration. Some energy is lost due to radiation of sound. Formal study of such a low damping material should be done under vacuum.

waveform, plot duration 7 sec. Frequency: 440 Hz. This contains thousands of cycles.
sound. All sounds are in wav format.
Polystyrene, tan delta = 0.02 inferred from the decay of vibration.

waveform, plot duration 0.51 sec.
sound.
Wood, tan delta = 0.03 inferred from the decay of vibration.
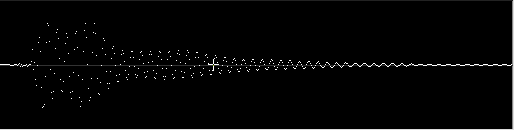
waveform, plot duration 0.185 sec.
sound.
PMMA, tan delta = 0.08 inferred from the decay of vibration.

waveform, plot duration 0.093 sec.
sound.
Magnesium alloy.

waveform, plot duration 5 sec. Frequency: similar to the aluminum fork (440 Hz) since the ratio of Young's modulus to density is similar to that of aluminum. This contains thousands of cycles.
sound.
Pure Magnesium.

waveform, plot duration 5 sec. Pure magnesium exhibits more damping than the alloy since the dislocations are more mobile. This sort of dislocation damping is nonlinear. The vibration damps out rapidly at first, when the amplitude is high. It damps more slowly later, when the amplitude is lower.
sound.
An expansion of the time scale by a factor of 32 shows the short oscillations corresponding to the natural frequency.
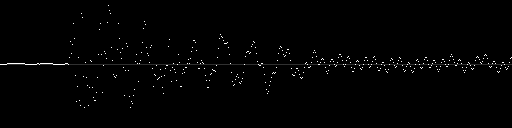
Brass.
Brass is dense. Its ratio of stiffness to density is less than that of aluminum or magnesium, therefore the pitch of the tone is lower. The tone continues for many cycles, so damping is small.

waveform, plot duration 10 sec.
sound.
Viscoelasticity: resonance structure
The broadband viscoelastic spectroscopy device in our lab can go from quasi-static to 100 kHz. A representative resonance spectrum of a solid polymer rod is shown in this diagram. If the end inertia is small, the resonances are in ratio 1, 3, 5, 7, 9. The clarinet, effectively closed at one end, has such a resonance structure in the low register. Compare with the flute, which is effectively open at both ends, and has resonances in ratio 1, 2, 3, 4, 5. Damping of air column resonances in musical instruments is dominated by radiation of sound.
Viscoelasticity: research
Negative Poisson's ratio materials
Foam stretching animation,
Honeycomb bend mpeg video.
Further details.
Further demonstrations are provided in our class pages.